Chapter 9
Navigation of Orbiter
from MECO to the ISS - Rendezvous with the ISS
Using the Spreadsheet to Demonstrate Navigation from MECO to Rendezvous with the Model, State and Course
Computers
A database was created in the final topic of Chapter 8 for the state of Orbiter at MECO,
that is, its coordinates, altitude, velocity, bearings and mass.
The first topic of this chapter showed a chart of the altitude, for 8,000 seconds
along the sub-orbit, that Orbiter would follow if no thrust were employed after MECO.
The apogee of that orbit reached about 182 km at about 2,370 seconds after MECO.
It could be seen from the chart that the sub-orbit decayed after that time.
The first and second topics of this chapter developed the application of three
computers to the design of the Course Commands that would guide Orbiter from MECO
to Rendezvous. The first topic showed those commands in the context of the
Input Table of our spreadsheet and are shown again next:
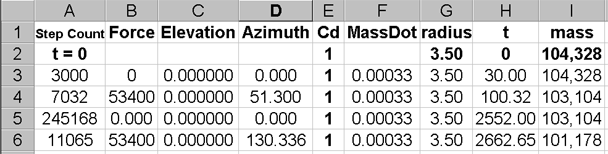
The table shows, in row 3, the 30 second delay after MECO in which Orbiter and the
External Tank are separated.
Next there is a 70.32 second burn that is intended to take Orbiter into the altitude
region of the ISS orbit. Such a burn could take place any time before the sub-orbit
intersected the Earth. Why then?
Common sense suggests that waiting until Orbiter was loosing altitude would waste
fuel and that the latest the first burn should happen is at apogee of the sub-orbit.
See a comparison of a burn 30 seconds after MECO with one 2,370 seconds after MECO
next:
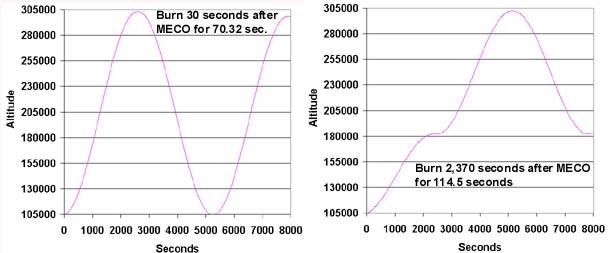
Delaying the first burn delays reaching ISS altitude and requires a longer burn
of the OMS engines, more fuel.
We had timed the launch from Kennedy to save fuel and arrive in orbit
quite close to the ISS. On the way to our objective we had employed Adaptive Conditional
Feedback to refine the Course Commands as they were executed so as to reach our
objective quite closely. It is those refined commands that are used in our spreadsheet
Input Table to derive various aspects of the mission.
The table shows the coasting interval of 2,451.68 seconds that takes Orbiter to near the
apogee of the first burn. The final burn of 110.65 seconds takes Orbiter
into the
ISS Orbit quite close to the ISS.
Launch may not occur Exactly When Scheduled
Note that the total time taken to reach the ISS from MECO can be adjusted within
fuel constraints by choices such as: choosing the time after MECO at which to initiate
the first burn; using some radial burn time versus tangential burn time for the
OMS engines in gaining altitude; choosing intermediate orbit altitudes a little higher or a little lower
than that of the ISS; trading burn time with burn force when using the RCS.
We do not model the final navigation process that employs the manual controls of
the RCS for docking.
See the, expanded, altitude and velocity results of the final, circularizing, burn
next:
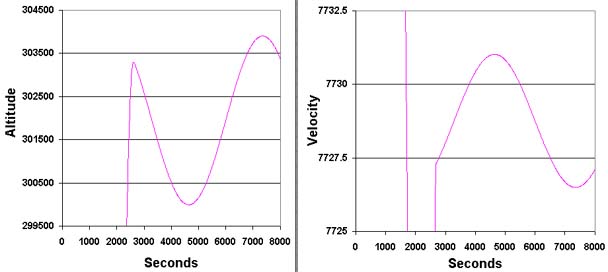
The controllables given to the solver in our 3D spreadsheet were the durations and
azimuth angles of the two burns and the duration of the coasting period between
the two burns. The objectives provided to the solver were apogee and perigee altitudes
of the ISS and the inclination of the ISS orbit.
The Hohmann Transfer Orbit and Navigation from MECO to the ISS
The Homann transfer orbit
is an idealized orbit maneuver using
two impulses of thrust that move a space vehicle
in a vacuum from one
circular orbit in a plane
to a second circular orbit in the
same plane. It consists one half of an elliptic orbit that connects the two circular
orbits.
Our illustration of navigation from MECO to the ISS took Orbiter from an
elliptic
sub orbit to the elliptic ISS
Orbit in an atmosphere using two non-impulsive burns.
In a practical situation needed corrections to the burns, their durations and their thrust
directions would be made by our postulated Adaptive Conditional Feedback
Control System.
Extending the Observation Time
Had Orbiter not docked with the ISS, it would circle the Earth in its own slowly decaying
orbit. The path taken by an undocked Orbiter is shown on a projection of
a selection of Earth's major cities next:
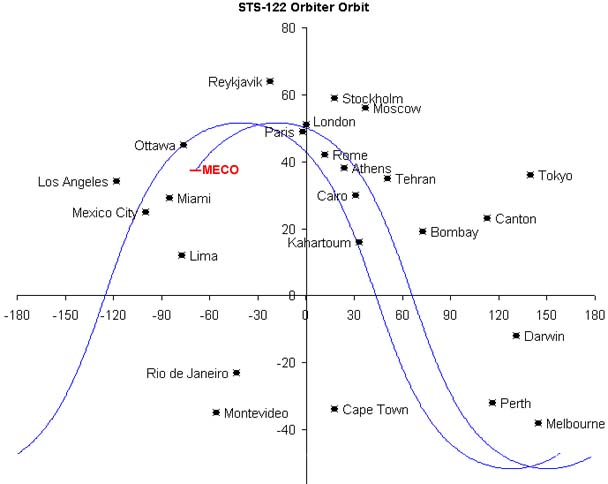
The path taken by the space station should differ slightly as it has a different
mass, cross-section and drag coefficient.
Energy from MECO through ISS Orbit
The varying kinetic and potential energy, and their total, of Orbiter as it ascends
from MECO into orbit is shown next:
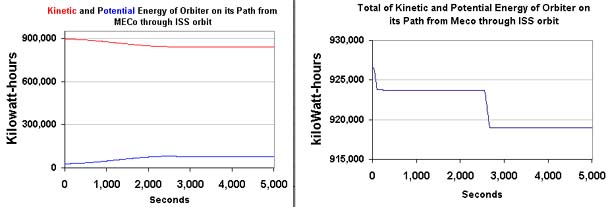
It is seen
in the left panel that potential energy rises and kinetic energy falls as altitude
is gained.
In the right panel it is seen that the sum of potential and kinetic energy
of Orbiter falls as it proceeds towards its orbit and the sum is seen to fall most rapidly during
the burn intervals.
The total energy dropping during burn intervals might seem counter-intuitive
but the reason for the energy drop is that the simulation calculates only the energy
of the Orbiter. When Orbiter uses fuel to heighten its orbit, it ejects mass at
high speed in the form of rocket exhaust.
Recall that potential energy is proportional
to mass as well as height.
The
potential energy of Orbiter
is reduced due to the use of fuel to gain height. The potential energy gained
with height is more than offset by the loss in potential energy due to mass
reduction.
A residual loss in energy between orbital maneuvers is
caused by air resistance.
Comment on the Demonstration
Today's missions to the ISS may not proceed as well as did our mission. It
may be necessary for Orbiter
to go into a lower orbit so as to have greater velocity to catch up to the ISS.
Alternatively a higher orbit might be needed to allow the ISS to catch up with Orbiter.
A Lower Orbit
Suppose that at MECO it is determined that if Orbiter proceeded to ISS orbit it
would likely arrive there about 10 seconds behind the ISS and an intermediate lower orbit
is chosen.
For an ISS orbit at 302 km and corresponding velocity of about 7728.7 metres per
second ISS would be about 77 km ahead of Orbiter.
In a 299 km orbit the velocity is about 7730.5 metres per second. In this orbit
Orbiter could catch up and transfer to the ISS orbit in about 12 hours.
For interest, the altitude and velocity for over a 12-hour period in a near circular
299 km orbit is shown next:
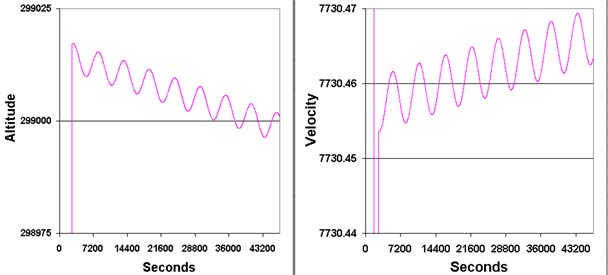
Getting
it right the first time and not requiring the use of an intermediate orbit
would be a good thing for the astronauts.
Summing Up Hands-On Math
Hands-On Math has been about exploring with mathematical models and through exploring
gaining a deep understanding of the use of mathematics and of the space that we inhabit.
Physical highlights have been the modeling of the effects of gravity, buoyancy and
resistance in our atmosphere, the art of ballooning, projectile behaviour and the
behaviour of rockets
in space.
Mathematical highlights include the techniques of a Solver to iteratively and
closely find unknown values given known or objective values. With solver
the absence of neat readily found mathematical solutions has not been an obstacle.
Procedural highlights include the use of Adaptive Conditional Feedback in a control
application.
Without that much said about it, at the core of the mathematical procedures has
been the use of numerical integration to solve awkward differential equations to
get answers that make real world sense.
Great value and satisfaction is obtained when the individual constructs his own
model. This is the reason that the spreadsheet was adopted for the modeling.
It has been the objective of the writers to provide sufficient information to the
reader for him to create the spreadsheet models.
For readers who choose not to construct some of the models, there is second hand
experience
to be gained by closely following the examples and, in some cases, using the two
calculators
that accompany Hands-On Math.
The spreadsheet version of one of the more complex calculators is provided as a download.
Calculators are provided with Hands-On Math so that the reader may carry out explorations
of his own fancy with Gravity, Ballooning, Atmospheric Resistance and Projectiles
without having constructed his own spreadsheets.
The complexity of the model of the three-dimensional rotating Earth
and its atmosphere and its various forms ruled against providing user-calculators in
that case. Considerable and, in the opinion of the authors, sufficient information about three-dimensional
modeling has been provided in the text for a reader
to construct his own three-dimensional
models.
HOM provides a great many web references for further on-line exploration of the
topics.
Last but not least, HOM has provided reference to many of the contributors and their
contributions to Mathematics, Science and Engineering that has brought mankind to
the verge of space.
Something for the Viewer to Ponder
The reader may have noted earlier in this topic that less fuel was required to reach
ISS altitude from MECO in the case where the first burn occurred sooner rather than
later.
Combine this observation with the current practice of having Orbiter and visiting
Supply vehicles use their engines to boost the orbit of the ISS.
Would less fuel be required to be raised to orbit, with correspondingly lower mission
costs, if Orbiter and the other vehicles just transferred boosting fuel to the ISS
so that it could use its own engines for boosting itself on a much more frequent
basis and thus experience much less loss of altitude between visits?
Finally
The reader may wish to see the views of the authors regarding considerations that
should apply to the further exploration of space by man. These views can be
accessed via Postscript on the upper row of navigation tabs.