Background of Hands-On Math
Historical Modeling from 1931
The inspiration for this website was provided by a 96 year old educator, Dr. Arthur
Porter. He expressed his views on the importance of "Hands On Learning" in
a four-page hand written letter to this writer. A motivational excerpt is
provided following:
Recollections and reflections
relating to the educational potential of the Bush Differential Analyzer, (D.A)
This brief essay is intended
to provide some background to what is essentially a new approach to the teaching
of the calculus, notably differential equations, to engineering students. Traditionally,
certainly in schools and institutes of higher education, the subject has been taught
by mathematicians with minimal interest in real life applications. Consequently
many students in the applied sciences have had difficulty in appreciating the tremendous
relevance of the subject. They have been indoctrinated in the conceptual, albeit
beautiful, language of mathematics without really understanding its power.
The ideas I shall portray were first set out by Dr. Vannevar
Bush at MIT, who as early as 1931 suggested that the machine could be valuable in
education as well as resarch. The important fact is that that the machine
can be regarded as a mechanical dynamic model of a system or process. In operation
the behavior of the system variables, (e.g. position, temperature, voltage, etc.)
under varying circumstances can actually be seen. And most importantly the relations
between them thereby providing insight and perspective. In other words the process
of solving a differential equation can actually be experienced.
These comments resonated with the writer with
the exception that today's students might find more lifelong continuing value from
hands on learning experiences with today's immensely more capable and widely available "machine", the Spreadsheet. (The complete letter can be obtained from HOM's "Download"
tab.)
The Differential Equation Analyzer - Definition
and History
In mathematics, a differential equation
is defined as an equation involving derivatives or differentials. In mathematics
the solution of a differential equation is regarded as being effected when it has
been reduced to an expression involving integrals, whether the actual integration
can be effected or not.
The role of the differential equation analyzer, DEA, is to provide specific solutions
to differential equations by physically carrying out, as a model, the integration whether or
not there is an analytical solution.
The heart of a DEA is the ability to integrate, albeit approximately.
For the DEA, Dr. Bush at M.I.T. employed a continuously variable transmission, a
disk and wheel. See
The Differential
Analyzer, V. Bush,
The Journal of the Franklin Institute, Vol.
212, No. 4, October 1931.
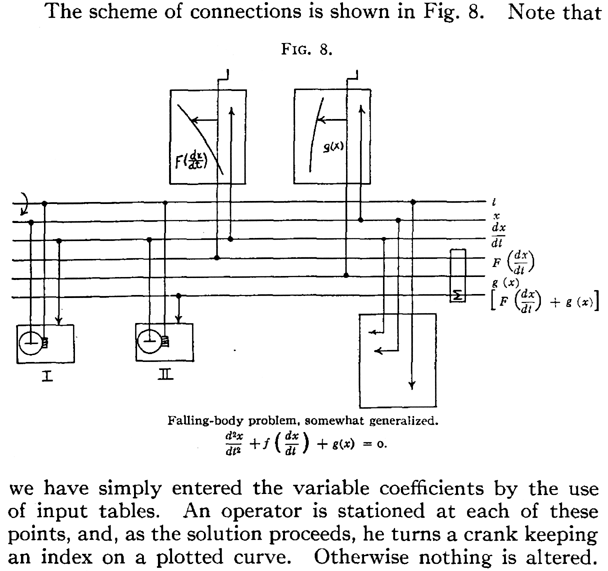
For the viewer's interest, the shaft connections etc., and some
text, for the Bush Differential Analyzer as set up for case of non-linear drag and
g force, excerpted from the 1931 article, are shown next.
-------------------------------------------------
---------------------------------------------------
No solutions are provided in the Bush paper as the focus was on the DEA.
Note that days of work are sometimes required to set up gear ratios etc., to scale
the device for a solution with the parameters required by a specific problem.
More history of the DEA is available on this site for downloading. Of special
note is, the 1935 paper "An Approximate Determination of the Atomic Wave Functions
of Chromium" by A. Porter. The work was done on the model differential analyzer
at the University of Manchester.
There is an excellent 2004 essay in PDF format " On the Development and Use of Differential
Analyzers" by
Dominik Schultes.
Also see:
C. W. Gear and R. D. Skeel. The development of ODE methods: a symbiosis between
hardware and numerical analysis. In A history of scientific computing (Princeton,
NJ, 1987), ACM Press Hist. Ser., pages 105-115. ACM, New York, 1990. A
.PDF file of the complete text can be found
here.
Modelling and Computing Ability both Expand as Time
Passes
There is an excellent portrayal of the side-by-side development of models and computation
in the 243 page, .PDF document, provided by NASA,
Black Magic and Gremlins. There, a history of the development of the
Flight Simulator where the development of computers and computation is intertwined,
is shown.
See an excerpt next:

The Spreadsheet, A Newcomer
Microsoft® Corporation's "Excel2000", a part
of their Office Suite, is the spreadsheet used throughout Hands-On Math. Excel
was used not only for modeling but also to construct drawings, graphs and spreadsheet tables and to produce
draft text.
There is much similarity in the operations that different spreadsheets perform.
For more general information, search "spreadsheets" on the net.
Although extremely capable and requiring only modest programming ability from the
user, the spreadsheet, seemingly lightning fast on small problems, is much slower
than the higher level programming methods employed for more demanding tasks. There
is little use of the spreadsheet for the heavy number crunching needs of today's
Science frontiers. Its main roles are in Science-Education and in Engineering.
There is the reward of a deep understanding that will come from your own use of a spreadsheet to model and explore topics in Science, Mathematics and Engineering.
The spreadsheet affords individuals the opportunity
to explore, play with, experiment on, vary, emulate, do a "what if" and to model
complex scenarios with the view of enhancing their own
personal understanding.
At the time of opening HOM to visitors, Microsoft
had announced their intention to provide free access and workspace on the web for
shared Excel spreadsheets. See
"Coming soon".