Chapter 8
Geostationary and
Planet Orbits
General Description of a Geostationary Orbit
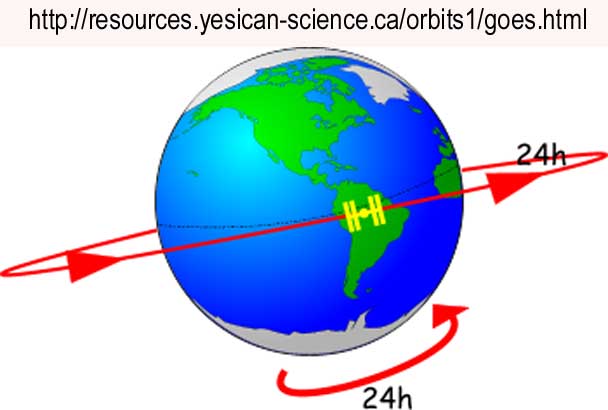
A geostationary satellite has an orbit that lies within
the Earth's equatorial plane. The shape of the orbit is circular and the angular
velocity of the satellite in the orbit matches the rate of rotation of the earth.
Viewed from Earth, the satellite would appear stationary in the sky. Since orbital
period is proportional to orbital altitude, there is only one specific altitude
that
can be used by geostationary
satellites. The geostationary orbit is said to be geosynchronous. See illustrative
drawing next:
Geostationary orbits are useful mainly for communications.
The altitude for these satellites turns out to be quite high, approximately
36 * 106 m. Accordingly, the delay of
radio signals to and from the satellites is large compared to that of paths using
either land-based facilities or near-Earth orbiting satellites. Such delay is unsuited
for transaction-oriented communications that rely on a rapid exchange of two-way communications.
The satellite does have a large view of the Earth. Consequently, geostationary
satellites are used predominantly for one-way broadcast service where the signal
transmitted to Earth would cover a large geographic area. International agreements assign space
in the orbit so that the satellites do not interfere with one another and global
coverage is satisfied by parties wanting to use the technology.
Geostationary orbits are not achieved precisely in practice and the satellites therefore show a small
amount of wander. Also, perturbations
from
such effects as the gravitational pull of the Sun and Moon cause such a satellite
to wander ever further from its assigned position. Before the wandering can go
too far astray, small rocket jets are used to perform orbital maintenance by nudging the
satellite back to within its proper space.
Orbit Parameters for Geostationary Orbit
Celestial Mechanics
provides the relationship:
P2 = [4*π2/G*M]a3 ,
where P is the period of an elliptic orbit,
a is the average of the apogee and perigee distances i.e.
the
radius r of a circular orbit,
and G*M is the standard gravitational parameter discussed
in the second topic of Chapter 5.
The foregoing relationship has the importance that it can be used to calculate G*M,
given the P and a of an elliptical orbit.
Our current use of the relationship is find r for a circular orbit given P.
Rearranging the relationship we find:
a = r = (P2 * G*M / (4 *
π2))
1/3
The determination of orbital parameters for a geostationary
orbit is straightforward. The orbital period, P, is the time for one complete revolution
of the Earth on its axis which is called a sidereal day and is 86,164.09054 s, more
or less. The altitude
of the orbit is the radius of the orbit minus the Earth's radius.
r
= (86,164.090542 * 398,600,441,800,000
/ (4 * π2))
1/3
= 42,164,169.6371354 m
Alt = r - earthRadius = 42,164,169.6371354 - 6,371,010.00
= 35,793,159.6371354 m
Orbital velocity is the tangential velocity of the circular
orbit and is such that one revolution is completed in one
sidereal day. It is the same value everywhere along the orbit, thus we can equate
the tangential velocity to the orbital insertion velocity, launch velocity as used by the spreadsheet:
Launch velocity = tangential velocity = distance / time
= 2 * π * r / P
= 2 * π * 42,164,169.6371354 / 86,164.09054
= 3,074.6600990408 m/s
We will assume the orbit is such that at t = 0, the Latitude
and Longitude of the satellite are both 0.0. Therefore:
Latitude, Longitude, Altitude = 0.0, 0.0, 35,793,159.6371354
The Elevation must be 0.0 degrees to specify a strictly
tangential velocity (no radial component). The inclination of the orbit is the angle
of the plane of the orbit measured from the equator. Since Azimuth on the worksheet
is measured from North and the satellite travels due East, we have:
Elevation, Azimuth = 0.0, 90.0 degrees, respectively.
The altitude is sufficiently high that a drag coefficient, Cd =
0.0 can be assumed. The orbit is not dependent on the mass of the satellite
since its mass is negligibly small compared to that of the Earth.
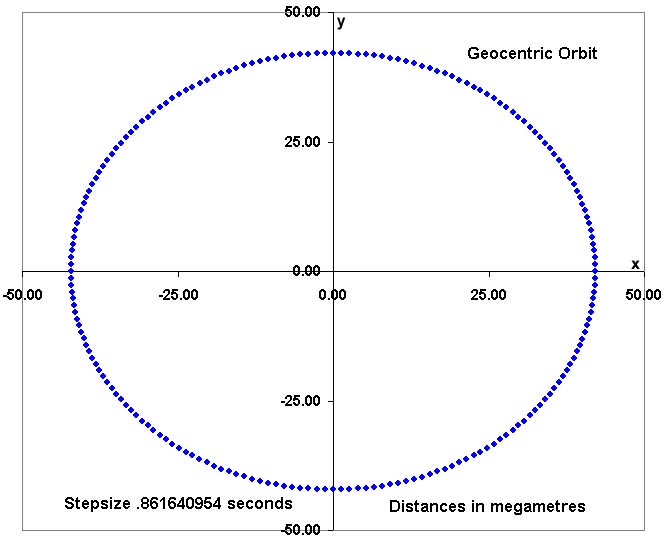
To get one complete orbit, set up the integrations with
a Step Size corresponding to a fraction (1/100,000) of a sidereal day. With a Steps
per Output = 500 and Number of Outputs = 200 we can get exactly one sidereal day
of orbit, that is one complete orbit.
A typical plot is shown in the following figure and as
expected the plot indicates a circular orbit.
Planetary Orbits
The same laws of physics that govern the motion of satellites
in orbit around the Earth apply to planets that orbit the Sun. For the purpose of
calculating planetary orbits, a slightly different spreadsheet is used. This allows
for certain parameters to be used differently than in the case of Earth orbiting
satellite spreadsheets.
The planet Earth orbits the sun in a plane called the
ecliptic. The ecliptic plane is analogous to the equatorial plane of the Earth except
that
we are dealing with the Earth orbiting the Sun in the ecliptic plane rather than
a geostationary satellite orbiting the Earth in the equatorial plane. Other planets
have orbits that are slightly inclined to the ecliptic. If we wanted to examine
their orbit, we could set the azimuth of the orbit to a suitable value that reflected
this inclination but we will limit our illustration here to that of Earth's orbit.
Latitude is used to specify a launch point not in the ecliptic plane
but we leave this at 0.0 since we will explore orbits in the ecliptic plane.
In the case of Earth satellites we use Altitude (distance above
the surface of the Earth) but for planets, it is more convenient to specify centre-to-centre
distance between the Sun and the planet, so instead of Altitude, we specify Distance
which means centre-to-centre distance for Sun to planet.
The Elevation and Azimuth are used to specify the angle
of the planetary orbit at its starting point. We will assume that the planet starts
at perigee and is in the ecliptic plane. For planets in our
solar system, the orbit is oriented such that the planet travels counter clockwise
when viewed from above (looking down on the North Pole of the Earth) therefore, Elevation = 0.0 and
Azimuth = 90.0.
Planet Earth Orbital Parameters
It is interesting to look at the orbit of Earth around
the Sun. We can look at the details of the orbital characteristics and relate them
to some observations that can be made from Earth. For the Earth's orbit, the parameters
we use are summarized below:
Latitude = 0.0
Distance = 147,090,000,000 km (Earth to Sun perigee distance)
Launch Velocity = 30,290 km/s
Elevation, Azimuth = 0.0, 90.0 (to place orbit in equatorial
plane)
G*M = 132,712,440,020,000,000,000 (this is the value for the
Sun)
Planet Earth Orbital Characteristics
The following figure shows the orbit for Earth with the
parameters set to values discussed in the foregoing paragraphs.
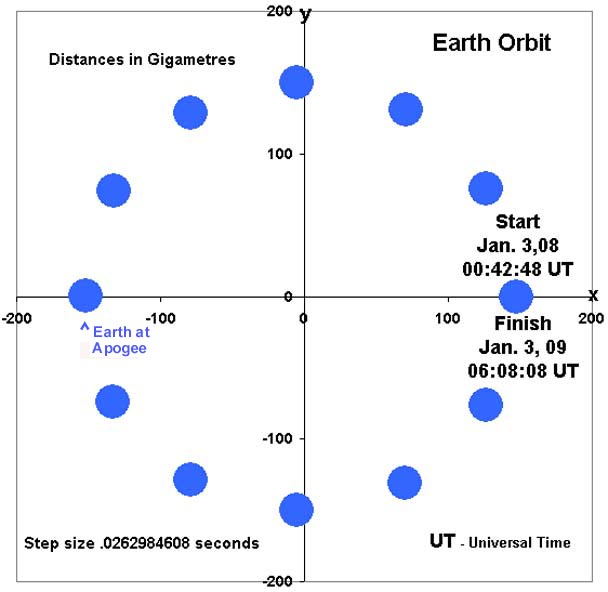
As can be seen, the Earth's orbit is very nearly circular.
Eccentricity is a measure of how far from a circle the orbit is. With an eccentricity
of 0.01688 the apogee distance is about 3.4% greater than the perigee distance.
The variation in the rate at which the Earth orbits the Sun
can be seen in the above diagram. Note that at one quarter of a year away from perigee, the earth
is more than halfway closer to the apogee. This is to be expected since the portion
of the orbit around perigee has the Earth traveling at its fastest.
Although the variation from a circular orbit is small,
the consequences of the elliptic nature of the orbit are easily observed on Earth. One
of the principal observations is the variation of the transit of the Sun across
the observer's meridian (the line that goes from North to South Pole passing through
the observers position). If the Sun moved at a uniform rate through the sky, this
passage would occur at the same time each day (at 12 noon in the centre of a time
zone). But because the Earth travels faster at perigee (during winter in the Northern
Hemisphere) than at apogee, it rotates around the Sun at a faster rate during this
time.
The effect is easy to see on the output of the integration for the orbit.
At perigee, the sun reaches the meridian before 12 noon while at
apogee position it does not reach the meridian position until after 12 noon.
For the former position the sun is said to be "fast of the clock" and for the latter
it is said to be "slow of the clock".
Clocks on Earth always mark the same
number of seconds between 12 noon on successive days (86400 s). As a consequence
of the higher rate of rotation around the Sun near perigee, at 12 noon from day
to day the sun appears to be further and further past the meridian. As the Earth
approaches apogee, it slows in its orbital velocity, the effect reverses, and eventually
the Sun will appear to cross the meridian before 12 noon. This cycle repeats from
year to year. The terms used for this effect are "sun slow" when it transits after
12 noon and "sun fast" when it transits before 12 noon. Through the year, the magnitude
of the effect amounts to about 15 minutes slow to 15 minutes fast, a total variation
of about 30 minutes.
Information About Time
In the above discussion, we examined Earth's journey around
the Sun and its rotation on its axis. These cycles have been used to define time
and its duration for most of recorded history. In the last half of the 20th century,
methods for the precise measurement of time showed that the rate at which the earth
rotates on its axis and other astronomical phenomenon such at the solar year were
irregular in duration. These irregular and unpredictable perturbations are in addition to
the regular ones such as discussed above which are due to the elliptical shape of the Earth's
orbit. Factors that cause such irregularities are the tidal friction on the Earth due to the Moon, Sun, and to a lesser extent other solar system objects,
as well as core-mantle interaction and plate tectonics causing shift in the mass distribution
of the Earth.
In 1967, the SI (Syste`me International) second was defined
as 9,192,631,770 periods of the radiation corresponding to the transition between two
hyperfine levels of the ground state of the caesium 133 atom. This duration of a
second was chosen to correspond to the duration of one second based on ephemeris time, the time
based on planetary motion, to the precision of measurement available. Further developments
have spawned a large number of time scales that are designed
to run at a rate based on the SI second.
For example: Barycentric Coordinate
Time (TCB) is the time base located at the centre
of mass of the solar system, but without
the solar system present; Geocentric Coordinate Time (TCG) is the time base for
the centre
of mass of the Earth without the Earth being present. The reason for removing
mass bodies in these two definitions is to remove an effect predicted by the Theory
of Relativity. This effect manifests itself as a slowing of the clock rate for a clock
that is close to a massive body, and therefore in a stronger gravity field, as
observed by someone further removed from the massive body. The gravitational
effect is
substantial. A caesium atomic clock running at the surface of the Earth will lose
about 22 ms per year relative to TCG due to the presence of Earth's gravity. In
fact, the Global Positioning System (GPS) is designed to account for the difference
in gravitational field (and hence clock rate) at the satellite altitude relative to observers
at sea level. This is necessary since these satellites beam down precise time of
day information that would otherwise drift and make position calculations inaccurate
should the difference in time be allowed to accumulate.
Coordinated Universal Time (UTC) has been defined for
the purpose of maintaining civil time, the time we use on our watches and other
timepieces. UTC is based on caesium clocks and can be accurately maintained to run
at the SI rate, but our civil clocks need to keep pace with the daily
motions of the solar system so that the Sun, for example, is always highest at around
12 noon every day. Thus another time scale is introduced for use with timepieces:
Universal Time (UT also called UT1). Since UT keeps pace with the irregular motion
of the Earth, a process is followed
where leap seconds are added/deleted to/from UTC where
the difference between UT and UTC is kept to less than 0.9 s. The last leap
second adjustment (at this writing) occurred on Dec 31, 2005. The previous adjustment was seven
years earlier.
Next
Earth observation satellites such as Radarsat-1 and, more recently, Radarsat-2,
occupy Polar Orbits as are explored in the next topic.